For values of n>>1, the probability density for a quantum mechanical particle in a 1-d box oscillates very rapidly.

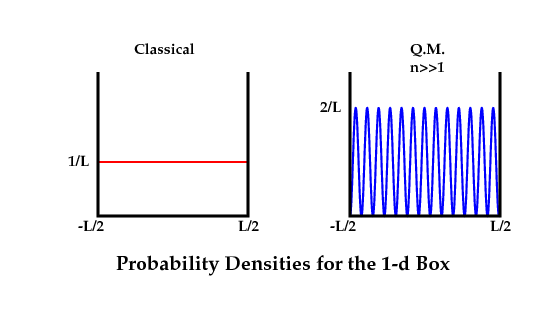
Thus, a calculation of the probability of detecting such a particle in the finite interval [a to b] involves integrating over several such oscillations. If this is the case, we may approximate the quantum mechanical probability density P(x) by its average value in the interval, namely,

P_avg(x) = Max[P(x)]/2.