The Matter with Dark Matter
Abstract
Over the decades the dark matter problem, if anything has
become more mysterious. The problem lies in that the existence of dark matter
is inferred solely from its gravitational effects. In recent years there has
been an alternative to dark matter for explaining these gravitational effects. Know
as Modified Newtonian Dynamics (MOND) it describes the motion of bodies in a
gravitational field by assuming that in the limit of small accelerations (
)
Background
The discovery by Zwicky (1933)
that visible matter accounts for only a tiny fraction of all of the mass in the
universe may turn to have been one of the most profound new insights produced
by scientific exploration during the 20th century. From observations
of the radial velocities of eight galaxies in the Coma Cluster, Zwicky found an unexpectedly large velocity dispersion, 
. Zwicky concluded that the mean
density of the Coma Cluster would have to be 400 times greater than that which
is derived from luminous matter for a velocity dispersion of 1000 km/s. Six
years later, Babcock (1936) obtained long-slit spectra of the Andromeda galaxy,
which showed that the outer regions of M31 were rotating with an unexpectedly
high velocity, indicating either a high outer mass-to-light ratio or strong
dust absorption. These observations indicated that the mass in the outer
regions of the Andromeda galaxy increased with distance from the center of the
galaxy, even though the optical luminosity of M31 did not. From these
observations Roberts & Whitehurst (1975) concluded that the mass-to-light
ratio had to be 
in the outermost
regions of the galaxy. This together with papers on the stability of galactic
disks by Ostriker & Peebles (1973), and that the
mass of a galaxy increases with increasing radius (Ostriker,
Peebles, & Yahil 1974), first convinced the
majority of astronomers that missing mass existed. Ostriker
& Peebles (1973) concluded that instabilities in galaxy disks could be
prevented by a massive spherical “halo” component (Bergh 1999).
By the mid 70’s the majority of astronomers were convinced
that unseen mass existed in the universe. However, it was unknown what form the
mass took, whether it was late M dwarfs, brown dwarfs, white dwarfs, black
holes, very hot gas or in some other unknown form. In the late 70’s it was not
clear that a paradigm shift (Kuhn 1962) would be required to interpret the new
observations that seemed to support the ubiquitousness
of missing matter in the universe. It had also been speculated that such a
paradigm shift might not be required if
Scientific Research
Estimates of the dark matter halo of galaxies are made with rotation curves. These rotation curves can rarely be measured beyond about twice the optical radius. Detection of the extended dark matter halo requires dynamical probes well beyond the optical radius. Satellite galaxies have provided a unique dynamical probe well beyond the optical radius of the central "primary" galaxy (Prada et al. 2003). While the inner parts of galaxy halos have density distributions that yield approximately flat rotation curves (example shown below), our understanding of the profile at larger distances is much poorer. The
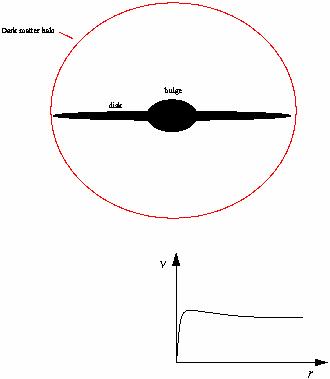
(Top) Three parts of a galaxy. (Bottom) Example of a rotation curve showing the radial velocity becomes constant at large radii
issue is critical because the
density profile that gives rise to a flat rotation curve (
) is different from that predicted by cosmological models (NFW
Navarro, Frenk, & White 1997) at larger distances
(
or 
- Newtonian). A method to probe the mass distribution at
large radii is to look at the velocities of satellites of a galaxy. However,
because the number of detectable satellites around galaxies outside of the
Local Group is small, one needs to study many galaxies to accumulate enough
statistics to study the profiles of other galaxies. As a result, observational
efforts to study the dynamics of satellites have been some what limited
(Erickson, Gottesman, & Hunter 1987; Zaritsky et al. 1993; Zaritsky
& White 1994; Zaritsky et al. 1997; McKay et al.
2002). Early results were obtained by Zaritsky et al.
(1993), Zaritsky & White (1994) and Zaritsky et al. (1997), who compiled and studied a sample
of about 100 satellites of nearby isolated spiral galaxies with an average of
1–2 satellites per primary galaxy. It was found that the line-of-sight velocity
dispersion of the satellites does not decline with the projected distance to
the primary galaxy. This result has been generally considered a strong argument
for the presence of dark matter at large distances (~200–400 kpc) from the galaxy center. Zaritsky
& White (1994) also found that the satellite velocity dispersion does not
correlate with the luminosity of the primary galaxy (Prada
et al. 2003).
Prada et
al. used SDSS data to plot the line-of-sight velocity differences of satellites
for primaries in the magnitude range -19.5 < MB < -20.5
(sample 1). This plot is shown below as well as one reproduced by me. The
dashed curve shows the r.m.s.
velocities for raw data with no interlopers removed. The full curve shows
theoretical prediction for equilibrium NFW halo with mass
.
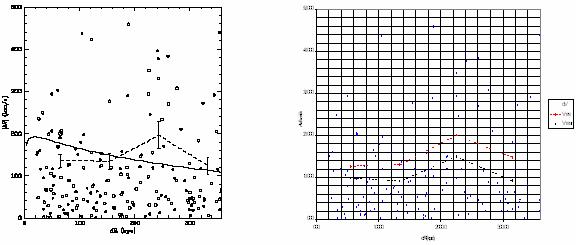
(Left) from Prada et al. (2003). (Right) my plot.
They also plotted a second sample with magnitude range -19.5
< MB < -20.5. This plot is shown below. We do not agree in
what the velocity dispersion should be at larger radii. The dashed curve shows
the r.m.s. velocities for
raw data with no interlopers removed. The full curve shows theoretical
prediction for equilibrium NFW halo with mass
.
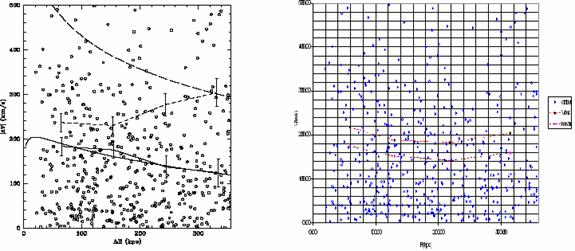
(Left) from Prada et al. (2003). (Right) my plot.
The reasons for the disagreement between plots is not known, which gives another reason that more work needs to be done on this method.
Expanding on the Scientific
Research
Deciding which galaxies around a primary galaxy are truly
satellites can be improved by knowing their morphology classifications. In the
past, most of morphology classification was done visually. In recent years the
vast amount of data produced by large surveys (such as SDSS, NED and 2MASS) has
made visual inspection a daunting task. One potential solution to this problem
is to automate galaxy classification using a neural network trained to
distinguish patterns and relationships using various photometric indexes. We
will present preliminary galaxy classification results using this method.
Reference
Zwicky, F. 1933, Helvetica Phys. Acta, 6, 110
Bergh, S. 1999, Astro. Soc. of the Pacific, 111, 657
Babcock, H. W. 1939, Lick Obs. Bull., 19(498), 41
Roberts, M. S., and Whitehurst, R. N. 1975, Apj, 201, 327
Ostriker, J. P., and Peebles, P. J. E. 1973, Apj, 186, 467
Ostriker, J. P., and Peebles, P. J. E., and Yahil, A. 1974, Apj, 193, L 1
Kuhn, T. 1962, The Structure of
Scientific Revolutions (
Milgrom, M., and Bekenstein, J. 1987, in IAU Symp. 117, Dark Matter in the Universe,
ed. J. Kormendy and G. R. Knapp (
Navarro, J. F., Frenk, C. S., and White, S. D. M. 1997, ApJ, 490, 493
Erickson, L. K., Gottesman, S. T., and Hunter, J. H. 1987, Nature, 325, 779
Zaritsky et al. 1993, ApJ, 405, 464
Zaritsky et al. 1994, ApJ, 435
Zaritsky et al. 1997, ApJ, 478, 39
McKay et al. 2002, ApJ, 571, L85
Prada et al. 2003,